poltbike.blogg.se - 2d shapes and 3d shapes

#2D SHAPES AND 3D SHAPES TRIAL#

Look for the following:ĭo they approach the task systematically or resort to trial and error?ĭo they get one view correct first, the top for example, before trying to get the other views correct? Ask them to work independently to create a building that matches the three views. Encourage students to share any other terms they use when talking about perspective (e.g. Use the language that is used on the slide - e.g “this is the top view”. Model constructing the building and viewing it from different perspectives. This could be done in pairs if necessary.Īfter a suitable time, ask students to compare their buildings and check that what they have built is correct. They will need a maximum of 10 cubes each. Give all students some cubes and invite them to construct the building.Slide 7 shows all three views simultaneously. Note that the drawings are what the camera sees and do not include cubes hidden behind others. Slides 4 – 6 show how plan views of a building can be created by lying it on a surface and viewing it from different positions. Students might spot iconic buildings or landmarks such as rivers or harbours. You could adapt the PowerPoint to include skylines from locations that are more relevant to your students (e.g. Ask students if they know the cities shown (New York, Melbourne, Shanghai). Slides 1-3 show some iconic skylines from around the world.

Use PowerPoint One to introduce cityscapes.In this session students create plan views (top, front, side) of models made with connecting cubes. Te reo Māori kupu such as raumata (net of a solid figure) and the names for different shapes could be introduced in this unit and used throughout other mathematical learning You could choose one context to frame all problems in, or vary the contexts presented in each problem. Students might also be motivated by interesting local buildings or iconic structures from around the world (perhaps from cultures that are relevant to their heritage). For example, traditional whare and fale were usually the shape of pentagonal prisms. Many buildings throughout the pacific are examples of prisms so are ideal for this unit. The contexts for this unit can be adapted to suit the interests and cultural backgrounds of your students, and to make connections with current events and relevant learning from other curriculum areas. scaffolding the process of representational drawing into a sequence of steps.manipulating the complexity of the 3-dimensional objects the students represent.

Tasks can be varied in many ways including:

providing opportunities for students to work in groups and pairs to encourage collaboration, peer-sharing of ideas, questioning, and reciprocal learning.

orientating cube models so the viewpoint matches the orientation of isometric paper.

providing access to sculpting materials, such as plasticine, so students can create their own 3-dimensional models.

altering the complexity of the shapes students are asked to construct.

This could include the use of digital cameras

explicitly modelling the building and drawing of shapes from various viewpoints, so students appreciate the minimal requirements of plan views.

providing materials, such as physical or digital models of 3-dimensional solids, so students can move and manipulate these, and themselves, in relation to the objects.

The learning opportunities in this unit can be differentiated by providing or removing support to students, by varying the task requirements. Nets are important in the packaging and construction industries. In this unit students explore creating nets which are flat patterns that fold to form a solid. A cone is similar to a pyramid in the same way that a cylinder is similar to a prism, as it has a curved surface, which means it is technically not a polyhedron. The figure below shows square-based and triangular based (tetrahedron) pyramids. square), and triangular faces that converge at a point, the apex. Pyramids have a base that names the pyramid (e.g.

$2d shapes and 3d shapes$

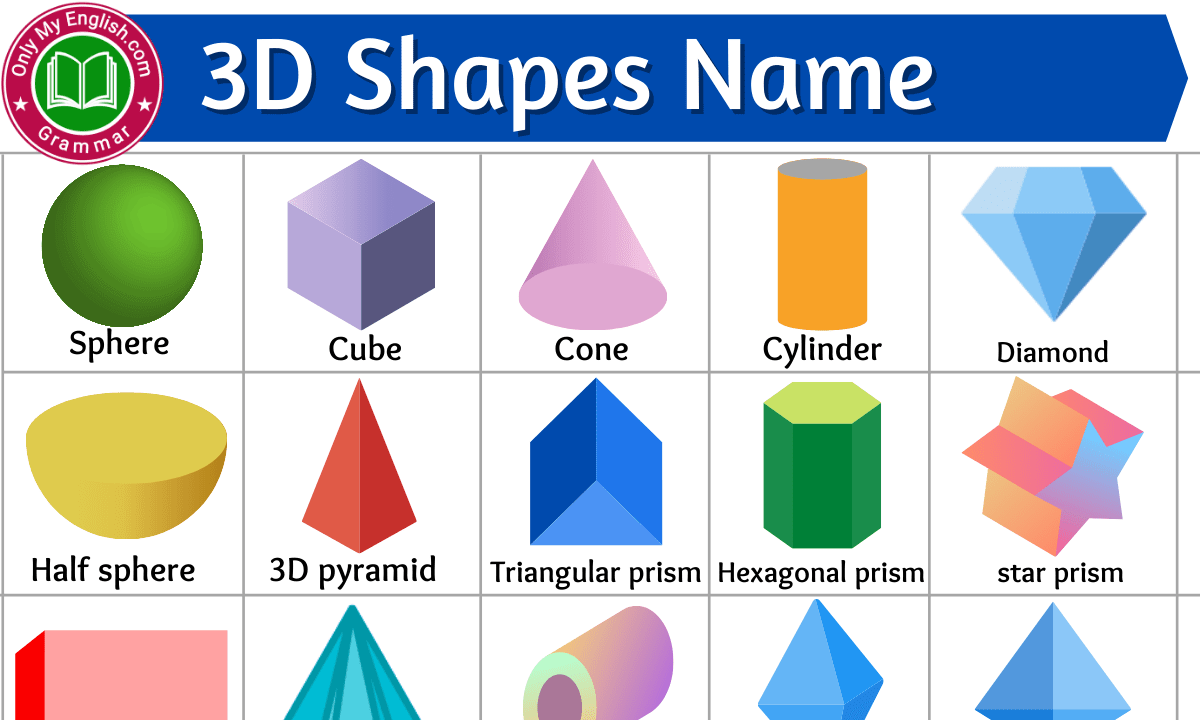
A cylinder is similar to a prism, however, because it has a curved surface, it is not technically a polyhedron. Having a constant cross-section is the defining property of a prism. In the figure below, the cube, cuboid, hexagonal prism, and triangular prism all have a constant cross-section if cut parallel to the end faces. Students learn about prisms and pyramids which are classes of polyhedra (plural). A polyhedron (singular) is a three-dimensional solid object that consists of a collection of polygons (flat shapes) that bound (enclose) a space. In this unit, students study solid (3-dimensional) shapes. These drawings are commonly seen in house design and usually show the structure from above (top or seagull view), front, and side. Plan views are different drawings of the same building.